This minimalist algorithmic piece is intended to be played before a Bohlen-Pierce concert or demonstration, to acclimate an audience to the general sound of BP pitches and harmonies.
It was generated using Java and the JMusic library.
Fuse Blue
This is a 12 bar blues I wrote using the Bohlen Pierce scale C D E F G H Jb A B C. The lead part is pedal steel and the other parts (except drums) were played on the Roland Handsonic. Recently remixed with real drums by my good friend Oscar Calderon.
BP on the Roland HandSonic HPD-15
The Roland HandSonic HPD-15 hand drum includes some tuned percussion instruments. You can edit the pitch of each pad in cents. The pads are grouped into 3 sets of 5, as shown:
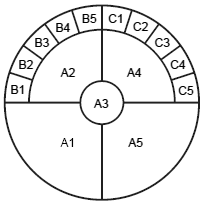
You can edit the pitch of each pad individually, and further adjust the pitch of an entire set (A, B or C). The pad set adjustment is very helpful when creating a patch for any HPD-15 instrument that is voiced for a higher or lower octave.
After several experiments, I finally settled on this arrangement of the Bohlen-Pierce scale notes. It is fully chromatic from D to D’. I didn’t include a low Db as it seemed less important than the high D’ for modal music.
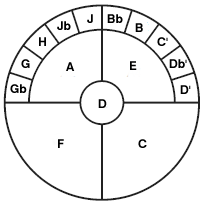
I tuned the notes to the C Lambda scale in just intonation with A=440 Hz (pad A2). The first song recorded with this system was Fuse Blue. The HPD-15 is somewhat buried in the final mix. I’ve created a separate MP3 file of just the HandSonic tracks so that you can hear what it sounds like:
I’m happy to answer any technical questions from other HandSonic players who are into BP or other alternate scales. The HPD-15 isn’t a real sensitive instrument, but its fine tuning capabilities make it a very useful tool for creating a variety of sounds in real time performance. I advise using a good frequency meter when tuning, as the readout of cents isn’t really very accurate. The tables below contain the fine tuning adjustments that I developed for several of the unit’s virtual instrument voices.
Continue reading
Sierra Pedal Steel
Most steel guitars can be adapted fairly easily to the Bohlen-Pierce scale. I made a fretboard from a piece of aluminum and some gold pinstripe tape. On this 12-string instrument, I configured the pedals and knee levers to the B Moll II mode (relative to J Lambda), which places the C Lambda mode nicely at the 4th fret. Here is the copedent chart:
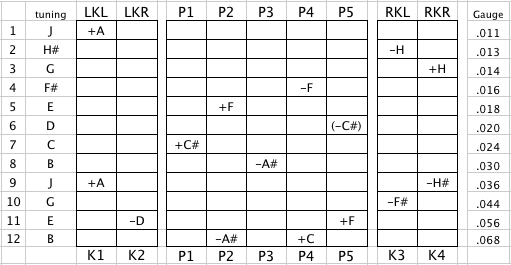
The 12-bar blues tune Fuse Blue was written and performed on this instrument:
While writing the song, I discovered that C Lambda sounds more bluesey if you flat the J note. The scale used is C D E F G H Jb A B C – a mode I haven’t seen documented anywhere. Background chords and the bass line were played on the Roland Handsonic. The drum tracks were generated by Band-in-a-Box (I got lazy).
The chords for this Fuse Blue’s pseudo I IV V progression are:
- I – C F A
- IV – E H A
- V – F G A
7-Limit Harmonies in the BP Scale
The most consonant harmonies in the Bohlen Pierce scale (to my ear) are those that fall within the gamut of Harry Partch’s 7-limit just intonation system. The BP intervals 9/7 and 7/5 (steps 3 and 4) seem to be the most pleasing, and their equal temperament counterparts are less than 4 cents from just.
The step 2 interval 25/21 is 302 cents, an acceptable minor third to western ears, but equal temperament changes it to a flat-sounding 292 cents.
The step 12 interval 25/9 (B note) is totally outside of the western 12-tone system.
This chart shows the intersection between the Bohlen Pierce scale and the 7-limit just intonation system. The D and B notes suffer the problems mentioned above. The difference between cents and round hundreds is a rough indicator of how alien the interval will sound compared to traditional western harmony.
| steps | name | ratio | cents | cents ET | 12-tone name |
|---|---|---|---|---|---|
| 0 | C | 1/1 | 0 | 0 | Unison |
| 2 | D | 25/21 | 302 | 293 | Minor 3rd |
| 3 | E | 9/7 | 439 | 435 | none |
| 4 | F | 7/5 | 582 | 585 | Tritone |
| 6 | G | 5/3 | 884 | 877 | Major 6th |
| 7 | H | 9/5 | 1018 | 1024 | Minor 7th |
| 9 | J | 15/7 | 1319 | 1316 | Dim 9th |
| 10 | A | 7/3 | 1467 | 1463 | Aug 9th |
| 12 | B | 25/9 | 1769 | 1755 | none |
| 13 | C | 3/1 | 1902 | 1902 | 12th |
Bohlen-Pierce Lambda Notes
This mellow piece selects notes at random from the Lambda subset of the Bohlen-Pierce scale (A=440 Hz). The sounds were generated by a Java computer program, using the JMusic library. The “instrument” is a sine wave.
The Bohlen-Pierce Scale
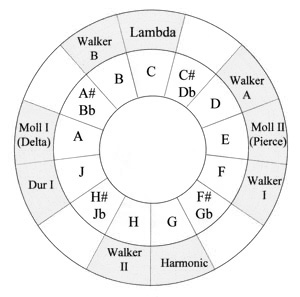
reference: www.transpectra.org
The Bohlen-Pierce (BP) scale is an alternative musical scale arrived at by dividing the harmonic twelfth (a 3:1 frequency ratio) into thirteen steps. The result is an unusual sequence of tones providing many consonant intervals and hence the promise of extensive musical possibilities to those willing to explore non-traditional sounds.
While the mathematics of the BP scale have been analyzed in some depth over the last thirty years or so, so far the body of music created on this basis is still small, and the field is wide open to exploration by composers.
Though both just and equal tempered BP scales have been postulated, the equal tempered version would normally be chosen for tuning instruments of fixed pitch. As with the conventional scale, when playing instruments of variable or partially variable pitch, notes are tempered by the player in order to bring intervals and chords into tune in a particular situation.
Each equal tempered Bohlen-Pierce scale step has a frequency ratio of the 13th root of 3, or approximately 1.088, corresponding to roughly 146 cents on the conventional 12 tone scale. Consonant BP intervals are mostly based on odd number ratios: 3:1, 5:3, 7:3, 7:5, 9:5, etc. Some of these intervals (3:1 = perfect twelfth, 5:3 = just major sixth, 6:5 = minor third, etc.) are familiar, while others are not heard, or at least not recognized as consonances, in conventional Western music. The octave (2:1) has no special status in this tuning system. Instead, the sequence repeats at 3:1, an octave plus a fifth. In BP terminology, this interval is called a tritave.
For present purposes, 440Hz (or 442Hz in some countries) has been chosen as the central reference pitch. On this basis, the pitches of the equal tempered Bohlen-Pierce scale, and their relation to the conventional 12 tone scale, are as given in the table below.
| Bohlen-Pierce Scale | ||
|---|---|---|
| frequency (Hz) | conventional pitch | BP note name |
| 16.3 17.7 19.3 21.0 22.9 24.9 27.1 29.4 32.0 34.9 37.9 41.3 44.9 48.9 53.2 57.9 63.0 68.6 74.6 81.2 88.3 96.1 104.6 113.8 123.9 134.8 146.7 159.6 173.7 189.0 205.7 223.8 243.5 265.0 288.4 313.8 341.5 371.6 404.3 440.0 478.8 521.0 567.0 617.0 671.4 730.6 795.0 865.1 941.4 1024.4 1114.7 1213.0 1320.0 1436.4 1563.1 1700.1 etc. |
C – 6¢ Db + 40¢ Eb – 13¢ E + 33¢ F# – 21¢ G + 26¢ A – 29¢ Bb + 18¢ C – 36¢ Db + 10¢ Eb – 39¢ E + 4¢ F + 50¢ G – 4¢ Ab + 42¢ Bb – 11¢ B + 35¢ C# – 19¢ D + 28¢ E – 27¢ F + 20¢ G – 34¢ Ab + 12¢ Bb – 41¢ B + 6¢ C# – 48¢ D – 2¢ Eb + 44¢ F – 9¢ F# + 37¢ Ab – 17¢ A + 30¢ B – 25¢ C + 22¢ D – 32¢ Eb + 14¢ F – 39¢ F# + 7¢ Ab – 47¢ A Bb + 46 C – 7¢ C# + 39¢ Eb – 15¢ E + 32¢ F# – 22¢ G + 24¢ A – 30¢ Bb + 17¢ C – 37¢ C# + 9¢ Eb – 44¢ E + 2¢ F + 48¢ G – 5¢ Ab + 40¢ etc. |
A (0) A# or Bb B C C# or Db D E F F# or Gb G H H# or Jb J A (1) A# or Bb B C C# or Db D E F F# or Gb G H H# or Jb J A (2) A# or Bb B C C# or Db D E F F# or Gb G H H# or Jb J A (3) A# or Bb B C C# or Db D E F F# or Gb G H H# or Jb J A (4) A# or Bb B C etc. |
Honey, Would You Get That?
(as posted on The Steel Guitar Forum)
I have a percussion instrument, the Roland HandSonic, that has 15 programmable touchpads. Last night I created a patch using a Gendér for all 15 pads, tuning them to the notes of the Bohlen-Pierce scale (as found on Wikipedia). There are some really ugly intervals in that scale, but some pleasant ones too.
So now I’m trying to compose something in BP that doesn’t sound like pure random crap. It’s pretty challenging. I’ll post a sound file when I’m further along with it. Why do I do things like this? Well, I believe that music is infinite in nature, and I like to explore things that reinforce that notion.
It’s not necessarily equal-tempered. I’m using the JI version of Bohlen-Pierce – because I can. 
update
Here are the sound files I promised. First the scale:
And here’s my little composition called Honey, Would You Get That? :